2
$\begingroup$

I know that many neurons have an input current-spiking frequency (I-F) relationships, e.g. as seen here.

However, all the I-F curves I've encountered show the input current in a fairly small range (few pA or nA), suggesting that there is no limit to this behavior.

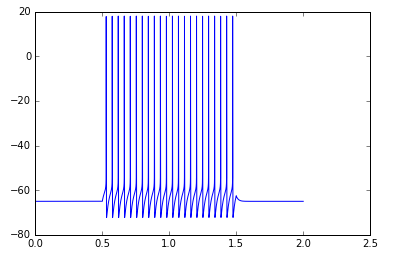
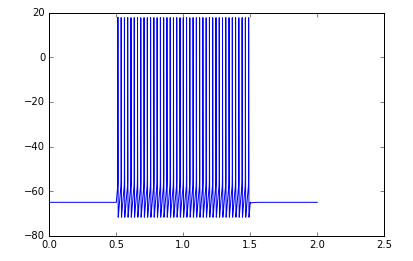
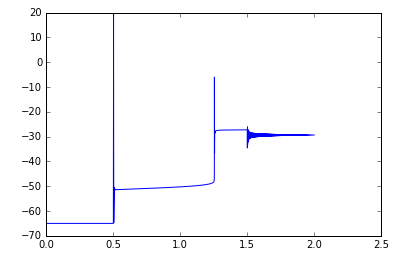
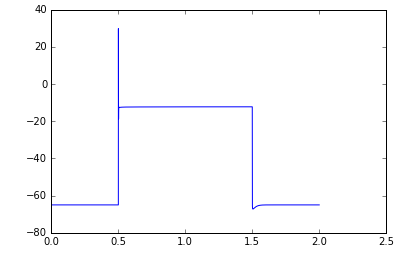
However, if the current is increased indefinitely, there is a point where the membrane voltage does not recover to the resting, or smaller, potential. For example, here are voltage traces of a simulated mitral cell:

At 0.1nA:

enter image description here

0.5nA:

enter image description here

1nA:

enter image description here

10nA:

enter image description here

How do neurons limit the amount of current that enters the cell? If the cell is large, with many incoming synapses, it seems like there would be stimuli that could result in "too many channels" open at the same time. How do cells ensure the input current remains within the acceptable range?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Current is Voltage (driving potential) times conductance. As the membrane potential approaches the Nernst potential of the conductance, the current approaches 0. Conductances can be turned on or off through receptor binding, but there is no such thing as voltage-independent current injection (at least under physiologically relevant conditions).

Indeed, it is the case that if you artificially inject large amplitudes of current into neurons, you observe voltage traces similar to your simulations. This will very quickly lead to cell death.

Improve this answer
$\endgroup$
3
  • $\begingroup$ so the current could still be high, but only for a short duration (<1 ms) before the membrane v reaches one of the Nernst potentials? Then the answer is that the Nernst potentials are what keeps the cell within the operating range. $\endgroup$
    – Justas
    Commented Jun 15, 2016 at 19:15
  • $\begingroup$ sure, if you want to say it that way $\endgroup$
    – honi
    Commented Jun 15, 2016 at 20:32
  • 2
    $\begingroup$ good point. In addition, there are homeostatic plasticity mechanisms that seems to control the overall gain if the firing rate is too high or too low. $\endgroup$
    – Memming
    Commented Jun 16, 2016 at 13:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.