25
$\begingroup$

Some intellectual, cognitive and perceptual tasks can be solved collaboratively. It is common knowledge that group performance is better than that of each single individual due to exchange of information (and without going into issues of motivation).

One widely known phenomenon is Wisdom of Crowds - when a group decision is much more accurate due to the averaging of individual biases. However, it is not the only possibility. Sometimes, a task can be divided into parts (and each participant works efficiently on his/ her own). Sometimes there are tasks too difficult to solve but easy to demonstrate that an answer is correct (and it suffices to have only one person in the group who answers correctly to make the group decision correct).

What I am interested in is, how the group performance scales with the group size? (The measure of performance can be error rate, time needed to complete a certain task or the deviation from the right answer.)

The question is motivated by one of my recent works, arXiv:1109.2044.

EDIT (clarification):

I am the most interested in low-level cognitive tasks (e.g. perceptual or linguistic), especially two-choice decisions (but also multiple choice, counting of blobs, solving a puzzle...). However, other quantifiable measures for cognitive task (including, say, time to complete a certain programming project vs number of programmers) are of my interest as well.

When it comes to the relation to my paper, I am curious if I miss an important reference or if my theoretical results can be supported (or undermined) by any existing experimental results (or even related in other context).

Improve this question
$\endgroup$
12
  • 2
    $\begingroup$ I like this question (+1) as do a lot of others. However, I wonder if at present it is still a bit broad. There are many possible group tasks that we could discuss. I wonder whether the question could be improved by focusing on a particular class of tasks (e.g., are you thinking about software project teams; tasks involving coordination; etc.)? $\endgroup$
    – Jeromy Anglim
    Commented Jan 23, 2012 at 5:47
  • $\begingroup$ @JeromyAnglim I'd definitely not go for a more localized question. $\endgroup$
    – Kris
    Commented Jan 23, 2012 at 11:20
  • 1
    $\begingroup$ @Kris I think that question on sleep is way too broad and multifaceted for the stack exchange format. The issue of scope seems like an interesting thing to discuss on meta; I posted a question meta.cogsci.stackexchange.com/questions/63/… $\endgroup$
    – Jeromy Anglim
    Commented Jan 23, 2012 at 11:50
  • 1
    $\begingroup$ @JeromyAnglim: I am the most interested in low-level cognitive tasks, esp. two-choice decisions (eg. perceptual or linguistic). However, other quantifiable measures for cognitive task (including, say, time to complete a certain programming project vs no of programmers) are of my interest as well. $\endgroup$
    – Piotr Migdal
    Commented Jan 23, 2012 at 13:16
  • 1
    $\begingroup$ @Piotr Migdal: What do you expect? For 2AFC tasks, your paper (which is nice, btw) summarizes the possible outcomes quite well, I guess. Depending on the knowledge about the group strategy, the model predictions should be quite precise. Hence, all you have to do now is asking the group which strategy they adopted. All empirical research that is left beyond your findings is the question which group strategy is adopted most often. $\endgroup$
    – H.Muster
    Commented May 21, 2012 at 13:31

2 Answers 2

Reset to default
12
$\begingroup$

It's a big topic. The relationship between group size and performance on a cognitive task is going to vary by several factors.

Here are a few thoughts:

  • The form of interdependence adopted by the group on the task will matter. When everyone can just work independently (e.g., taking calls in a call centre), then it makes sense that output would increase almost linearly with increasing group size, in the absence of motivation or learning issues.
  • In the context of project teams, software teams, and so forth, I imagine the issues would be a lot more complex and that in some situations increasing group size might even reduce performance of the team. There a few articles cited here that discuss this.
  • A classic study in the context, albeit from a motor task, of the relationship between group-size and group performance is that by Ringelmann (see the wikipedia article) that looked at rope pulling power as a function of group size. The basic finding was that average pulling power declined with group size. This has been interpreted as evidence of social loafing, but their are also coordination issues as well.
  • Some tasks are heavily influenced by the performance of the weakest or strongest member. For example, a musical group will often sound bad if only one member plays poorly. Or other tasks it will be strongest member, as you say if you have are playing at a trivia night, and you have one person with all the right answers on the table.
Improve this answer
$\endgroup$
5
  • 1
    $\begingroup$ The OP, however, is only interested in up-scaling the model. How do any of these factors influence the ease or level of difficulty in scaling up? $\endgroup$
    – Kris
    Commented Jan 23, 2012 at 11:21
  • $\begingroup$ If I understand your question then for the four dot points above the scaling would be: a. almost linear; b. increasing but possibly with an inverted u quadratic; c. monotonically increasing and monotonically decelerating; d. increasing or decreasing. $\endgroup$
    – Jeromy Anglim
    Commented Jan 23, 2012 at 11:31
  • $\begingroup$ @Kris but these are just some basic thoughts; I'm sure the question could be answered in many different ways; I'm keen to see what others have to say $\endgroup$
    – Jeromy Anglim
    Commented Jan 23, 2012 at 11:33
  • $\begingroup$ Thank you for the very useful info. I hope the OP finds this useful, too, in which case you could include it in the answer. I would wait know how each of the four are influenced. $\endgroup$
    – Kris
    Commented Jan 23, 2012 at 11:35
  • $\begingroup$ @JeromyAnglim: I am interested in collaboration on cognitive tasks, so only the part on software development is answering my question. I am aware of Ringelmann effect (however, it's more about motivation/management than anything else). And yes, for different tasks the scaling is likely to be different. $\endgroup$
    – Piotr Migdal
    Commented Jan 23, 2012 at 13:11
11
+50
$\begingroup$

I recently read a paper, which showed a mathematical model for performance scaling of research groups in different scientific branches. I'm aware you were originally asking for smaller "cognitive tasks" and project-like group-processes in the comments, but output and quality of publications/patents is probably anyway a better and more objective measure on a larger scale. Also, the paper shows that optimal group sizes in different academic disciplines varies strongly, so comparing, e.g. single groups in math and biology solving tasks is probably not very meaningful (Jeromys 1st comment) for your original question and only valid for exactly that discipline/distinct cognitive task, which would make a general analysis/conclusions tricky/impossible.

Assumptions:

The extent to which a group’s research quality is higher or lower than average is a function of many parameters such as the innate calibre, education and experience of its members, the quality of managerial support and of facilities and infrastructure, the impact of external and international collaborations, the prestige and confidence inspired by the institution and by past successes, etc. All of these parameters contribute to the quality of an individual group but, in general, research quality is size independent according to this argument. [...] However, it would be naive to retain this viewpoint alone because it neglects the importance of interactions between group members.

The model:

A group has $N$ members, therefore the total number of pairs of individuals along which channels of communication can open is

$N_{T}=N(N-1)/2$ (quadratical scaling)

So group quality tends to rise continuosly with quantity. The main statement of the article however is that the rise of quality is independent of group quantity after a group reaches a upper critical mass ($NN_{C}/2$ linear scaling) and increaes only with much smaller slope further, basically because a single researcher can only communicate meaningfully with a limited number of other researchers (sub-groups build within big groups, specialization)

Comparison with available data:

I will not copy paste all the data fits here, look them up in the paper, well worth and interesting, many academic disciplines are compared in different countries and match the model and quadratic scaling quite well in different academic disciplines.

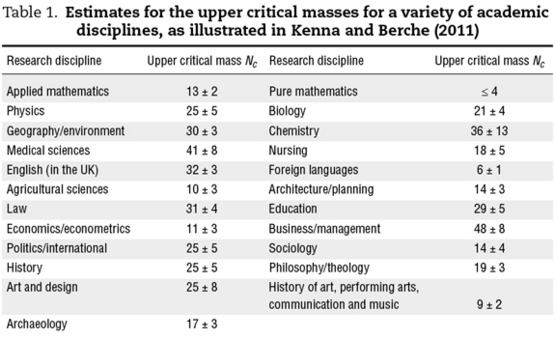
enter image description here

So the scaling for groups is the same, as mainly determined by meaningful face-to-face communication, the different critical mass among different academic disciplines has probably to do with the necessary amount of researcher you need to run, for example, a high-technology research laboratory (many students who master the single instruments) vs. mathematics (formalized language, not the broad spectrum of problems like in natural sciences) vs. humanities (reading and discussing a lot of literature)

Implications:

To improve overall research performance in a country, increase groups below the size of the critical mass instead of further increasing of already abnormal big research groups, where a slope for performance scaling dependent on $N$ still exists, but is much smaller than below the critical mass

Side note: Apart from this critical research group size I'm aware of a new trend in bigger companies to forbid or restrict e-mailing for the majority of employees, because only a marginal amount of this e-mails is really important according to studies (15% I rememember mentioned by Volkswagen AG, but not the source currently)

References
Kenna R, Berche B (2011). Critical masses for academic research groups and consequences for higher education research policy and management. Higher Education Management and Policy 23 (3), 9-29.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I am aware of that paper, and actually I like it very much (and had nice discussion with an author, and even used it as an example on academia.SE). However, scaling is quadratic/linear, depending on the group size. And discipline changes only critical group size, not the scaling properties (which may be more a subject of social interactions + some hidden factors correlating group size and a measure of performance). $\endgroup$
    – Piotr Migdal
    Commented May 21, 2012 at 13:00
  • $\begingroup$ But anyway, thanks and +1 :). (Though I clarified in the question, that I am the most interested in low-level task; a grade for the total research output, being a complex process by itself, is hardly low-level.) $\endgroup$
    – Piotr Migdal
    Commented May 21, 2012 at 14:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.