I have a background in physics and has enough knowledge in Fourier analysis, group & representation theory, projective geometry, complexity theory, and dynamical systems theory. What introductory textbooks on mathematical psychology should I read?
$\begingroup$
$\endgroup$
Add a comment
|
4 Answers
$\begingroup$
$\endgroup$
I also like https://bayesmodels.com/.
I posted the question on twitter, you could check out the responses.
Joachim Vandekerckhove suggested:
- Lewandowsky, S., & Farrell, S. (2010). Computational modeling in cognition: Principles and practice. Sage Publications. https://www.amazon.com/Computational-Modeling-Cognition-Principles-Practice/dp/1412970768
Edgar Kausel suggested the following classic that is still good to read:
- Coombs, C. H., Dawes, R. M., & Tversky, A. (1970). Mathematical psychology: An elementary introduction.
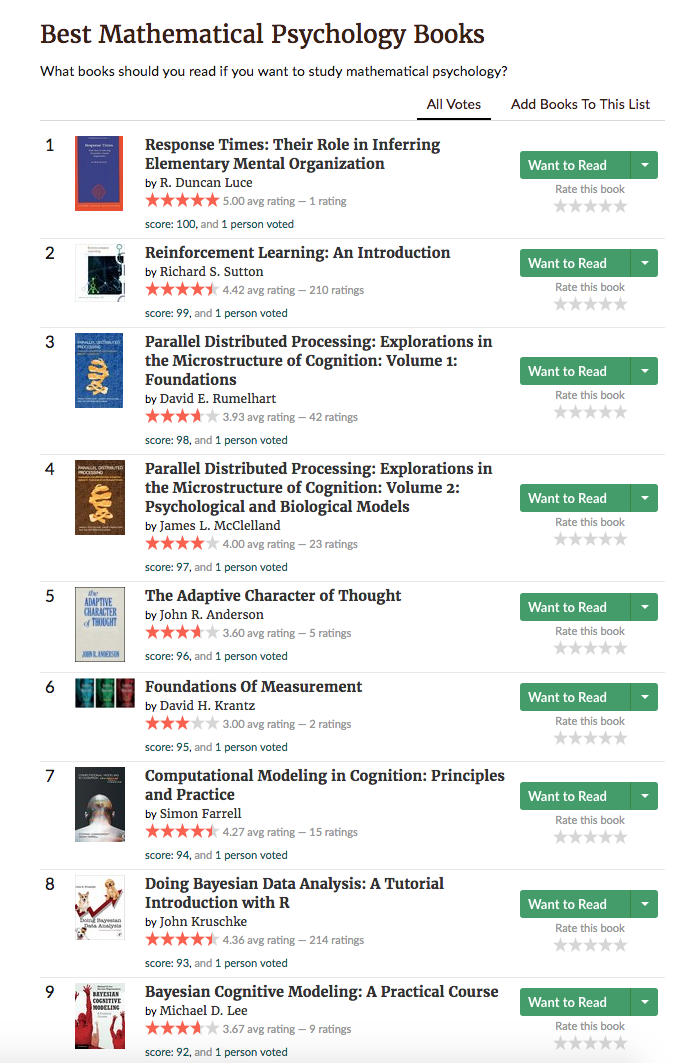
Daniel Litted mentioned his list that includes over 15 recommendations https://www.goodreads.com/list/show/121661.Best_Mathematical_Psychology_Books
Here are the first few:
answered Apr 20, 2018 at 2:14
$\begingroup$
$\endgroup$
3
I hold a Bachelor's in Applied Mathematics and, for my Master's in Neuroscience, I have used mainly the classic one from Kandel Principles of Neural Science. With regard to Mathematical Psychology I would suggest Oxford Handbook on Computational and Mathematical Psychology
answered Apr 19, 2018 at 16:27
-
-
$\begingroup$ It depends on what you are looking for. What I like in this book, is having a glimpse on how oversimplified artificial neurons usually are. And, even so, how incredibly powerful and useful they can be. $\endgroup$ Commented Apr 19, 2018 at 16:33
-
$\begingroup$ my bad, but I realize what I need is about mathematical psychology, not theoretical neuronscience. The two are really easy to be confused. Do you have a recommended book for that? $\endgroup$– OokerCommented Apr 19, 2018 at 16:36
$\begingroup$
$\endgroup$
I really like https://bayesmodels.com/ There's also a lot of fun you can have at http://probmods.org/ that feeds into a bunch of current cognitive modeling work, see also http://agentmodels.org/
You might enjoy "Complex probabilistic inference: From cognition to neural computation" by Samuel Gershman and Jeffery Beck.
With the background you describe, you probably want to jump straight into the deep end and get stuck into some journal articles pretty quickly. If you can find a BBS (Behavioral and Brain Sciences) target article close to your interests you'll get an instant overview from a diverse group of commentators, it's an awesome format. "Trends in cognitive sciences" also aims for pretty readable overviews (and they're a lot shorter!). You'll probably have to narrow down your focus a little to get mileage out of either of them though, 'mathematical psychology' is pretty broad.
$\begingroup$
$\endgroup$
Louis Narens has a theory of meaningfulness based on the axiom of choice and Erlanger program, which is a unification between projective geometry and group theory.
There is also a review on the whole field: Mathematical Psychology: Prospects For The 21st Century: A Guest Editorial, J Math Psychol. 2008 Oct; 52(5): 269–280.