I think there are 3 questions here:
(1) What are the equations of the the cone opponent colorspace? You answered the question yourself, it is literally additions and subtractions of the response of the 3 types of cones. Note that this is an approximation, more than 10 pathways composed of over 80 cell types have been identified. There are also some small controversies, like that it is unclear whether the S cone contributes to the luminance axis or not. This specific colorspace is called the DKL colorspace. It has been shown by Derrington, Krauskopf & Lennie (hence the name) to account extremely well for the activity of cells in the lateral geniculate nucleus (a relay between the retina and the primary visual cortex). However it is not a very meaningful colorspace perceptually speaking. It is a linear transformation of the LMS cones colorspace, thus as shown by Erwin Schrodinger they are indistinguishable.
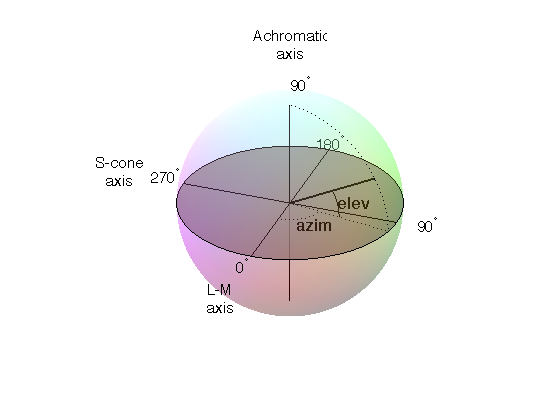
A depiction of the DKL colorspace from the psychopy documentation.
(2) Where are the rods? Well rods are not thought to contribute to color perception. A simple demonstration of that is that at low-lights levels (called scotopic vision) you lose the ability to discriminate colors. The reason is that rods are more sensitive than cones.
(3) How to relate a monitor RGB colorspace to the DKL colorspace? As I said the LMS colorspace and the DKL colorspace are linear transformations of each other. This is also true for the CIEXYZ colorspace, and any RGB colorspace. The LMS and DKL colorspaces are based on a standard observer from the CIE (Commission Internationale de l'Eclairage, a committee that fixes standards for anything related to visual displays): 2 sets of measurements of the cones sensitivity functions agreed so well that they were averaged and the result was decided to be a "standard observer". The XYZ colorspace is based on a somewhat arbitrary transformation of these spaces. In any case any of these spaces can be recovered from any of the others using a simple matrix multiplication. transformation matrices for standard colorspace (LMS, DKL and XYZ) are known and can be found on the internet. For a specific monitor you would need to measure its properties and compute it yourself.
Derrington, A. M., Krauskopf, J., & Lennie, P. (1984). Chromatic mechanisms in lateral geniculate nucleus of macaque. The Journal of physiology, 357(1), 241-265.
Schrödinger, E. (1994). On the relationship of four-color theory to three-color theory. Color Research and Application.
