3
$\begingroup$

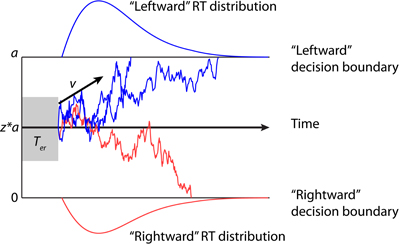
In sequential sampling models - for instance Ratcliff and Smith, (2006) - participants' responses in a binary choice experiment are modelled by a particle, which moves up or down towards the boundaries for selecting each response over time according to the evidence in favour of each, or, analogously, their expected utility (Busemeyer & Townsend, 1993), in a way that looks something like this:

enter image description here

My question is if anyone knows, and preferably can provide a reference for, whether in such a model responses are best predicted by

  • the difference in the evidence for/expected utility of each response (i.e. $P(Response\ A) = Evidence\ for\ A - Evidence\ for\ B$) or
  • the ratio of evidence/expected utility (i.e. $P(Response\ A) = \frac{Evidence\ for\ A}{Evidence\ for\ B}$)

My intuition is that it's the ratio between the two responses, rather than the absolute difference, which should best predict responses, but I can't find a reference for this, and I'm sure this question has been answered somewhere before.

Has anyone any ideas here?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

Unless I'm misunderstanding what you mean, ratio of evidence is actually a terrible method because that would end up with an immediate decision as soon as any evidence is encountered for either side, giving a ratio of infinity for that side (something/0 is greater than any finite decision threshold).

Try Gold and Shadlen 2007 for a review http://synapse.princeton.edu/~sam/gold_shadlen07_annu_rev_neurosci.pdf

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Partial credit (+1): the reference is a good one (thanks), but as I understand it, it suggests the ratio to be best predictor in principle: "The decision requires the construction of a DV [decision variable] from e [the evidence]. For binary decisions, the DV is typically related to the ratio of the likelihoods of $h_1$ and $h_2$ given e: $l_{12}(e) ≡ P(e | h_1)/P(e | h_2)$". $\endgroup$
    – Eoin
    Commented Nov 16, 2015 at 14:57
  • $\begingroup$ I think what you say about the case of $Something/0$ requires that participants begin each trial with a prior value $0$ for each option, which isn't true - unbiased participants should start with a prior of $.5$ on each option, and adjust this belief in response to new evidence. $\endgroup$
    – Eoin
    Commented Nov 16, 2015 at 15:01
  • $\begingroup$ nice. yes, ratio is only bad if you don't have a prior. $\endgroup$
    – honi
    Commented Nov 16, 2015 at 18:36
  • $\begingroup$ A benefit of the absolute difference method is that it becomes more likely that a decision will be made as time goes on (random walks tend to increase in range over time). This can be added to the ratio method by adding in an "urgency" value that decreases the decision threshold over time $\endgroup$
    – honi
    Commented Nov 16, 2015 at 18:40

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.